Assessment of the Potential of Tidal Range Energy in Malaysian Exclusive Economic Zone (EEZ) using Multi-mission Satellite Altimetry Data

Uti, M. N.,¹ Din, A. H. M.,^1* Yusof, N.,² Rasib, A. W.,¹ Hamden, M. H.¹ and Hashim F. E.³

¹Geospatial Imaging and Information Research Group (GI2RG), Faculty of Built Environment and Surveying,

Universiti Teknologi Malaysia, 81310 Johor Bahru, Johor, Malaysia,

²Tropical Resources Mapping (TropicalMap), Faculty of Built Environment and Surveying, Universiti

Teknologi Malaysia, 81310, Johor Bahru, Johor, Malaysia

³Faculty of Mechanical Engineering, Universiti Teknologi Malaysia, 81310 Johor Bahru, Johor, Malaysia

*Corresponding Author

Abstract

Since the first launch of the altimeter mission in 1978, it has been significant for ocean studies in many fields. Today, satellite altimeters enable users to use their products for resource assessment. The inadequacy of in-situ data in the coastal and offshore Malaysian Sea has deprived the tidal range resource assessment. The depletion of natural resources and climate change raised the need for sustainable energy. Vast ocean resources surround Malaysia and have the potential to harness tidal range energy. The altimetry mission can provide extensive ocean data in both spatial and temporal resolution. This research used the multi-mission satellite altimeter to estimate HAT and LAT for the tidal range generation and a tide gauge for validation purposes. A GIS approach is adopted to assess the potential location by mapping the resource and marine conflicts. Validation showed that the estimated HAT and LAT have RMSE values of 25.5 cm and 17.4 cm, respectively. Several locations, including the Pahang, Selangor, and Sarawak coasts, are identified, with a potential resource range of 55.25 to 129.33 kW/m². This research presents insight into Malaysia's tidal range energy potential, providing valuable information to stakeholders and the government for clean energy development.

Keywords: Geographical Information System, Highest Astronomical Tide, Lowest Astronomical Tide Ocean Renewable Energy, RADS, Satellite Altimeter, Tidal Range Energy

1. Introduction

The tidal range is the height difference between high and low tide. It is induced by the gravitational forces between the astronomical bodies of the moon and sun with the Earth's rotation and varies in time and place [1]. Generally, the sea level was continuously observed by the tide gauge installed at the coastal sites [2] for tidal datum computation, coastal monitoring, navigation, and marine conservation and preservation [2] and [3]. In Malaysia, tide information is acquired from the installed coastal tide gauge stations by the Department of Survey and Mapping Malaysia (DSMM). Until now, 22 operating tide gauges on the Malaysian coast provide continuous information about station location, tide level, mean sea level and harmonic constant. However, the tide gauge observations are point-based measurements and are limited to coastal areas because tide gauge installation in the offshore area is absent. Thus, the tidal range off the coast was only considered equivalent to coastal [2]. The first launch of the altimeter mission in 1978 improved the observation of global ocean. Altimetry mission provide global sea surface height data (SSH) that can be offered as tide gauge measurement to the offshore area [2].

There are several ways that tides can be used to produce electricity, such as tidal barrage power systems (dams), which make energy based on the difference between high and low tides [4].

While tidal stream power systems utilise ocean currents to power the turbines, they are most effective in locations around islands and coasts where the currents are swift [4]. Tidal range power is produced from the difference in tidal range between low and high tides. The discovery of tidal power can be a flashback to the early 6^th century when there was a tide mill in Strangford Lough of Northern Ireland [5]. A tidal range power plant's basic design involves impounding much water where a head difference can be produced and allowing water to flow into or out of this area through low-head hydrokinetic turbines [6]. According to Kai et al., [7], Malaysia's ocean characteristics are low kinetic energy-flux density, low current speed, low tide, and low water depth, making the tidal stream resources insignificant to the nation's energy mix. Even though tidal stream device installation is less expensive, more realistic and has fewer environmental impacts compared to tidal range technology [8] and [9], tidal range energy is beneficial to Malaysia since Malaysia has an extended length of coastlines that are reliable to harness the tidal range as an alternative energy source [10]. Besides, growing awareness of the enhancement of tidal power potentially supports shifting the use of fossil fuels in generating electricity [11]. Tidal power is a non-pollution energy production that can cleanly generate electricity. Past and recent studies have proved that Malaysia has the potential to harness the tidal range energy. A study by Samo et al., [12] found 18 sites on Sarawak coastlines were appropriate for tidal range energy extraction, including Sematan, Sibu, Kuala Lawas, etc., with two areas with the highest potential which is Tanjung Manis and Pending, Sarawak, with a possibility of 39.2 kW-50 kW and 25.1 kW-33.1 kW, respectively. A study by Samo et al., [11] found that Tawau, Sabah and Pending, Sarawak are the potential locations for tidal energy based on high and low tides with optimum power generated of 67.0 kW and 115.5 kW, respectively. The generated tidal range power by Samo et al. [11] is based on the tidal data that obtained from the Sarawak marine department, however, the result of potential location for Tawau, Sabah shown a data discrepancy due to the tidal data used in the study is only in the Sarawak region.

Aside from local tidal measurement from tide gauge, satellite remote sensing also provides observation to estimate the sea level by calculating the time travel of returned signal emitted from the radar altimetry [13]. The advantage of satellite observation is that this technique ensures broad coverage with repetitive and consistent observation for long periods, which is beneficial in accommodating long-term data for sea-level research [14].

According to Nehama et al., [15], satellite altimetry data provide a solution to the lack of in situ tide gauge data, which are essential for comprehending various marine processes worldwide. Altimetry sensor has proven their reliability in providing sea level data for various studies such as coastal risk assessment [15], tidal constituent derivation [16], and tidal energy resources assessment [17]. These have proved the reliability of altimetry sea surface height data to be used in tidal range estimation by analysing the important element for tidal range derivation, such as the tidal constituent derivation. Tidal constituent derivation from satellite altimeter by Hamden et al., [16] has shown a good RMSmisfit with a value within 10 cm. Thus, this research highlights the use of altimetry sea surface height data to derive the tidal range for estimating the tidal range energy inside the Malaysian EEZ.

1.1 Tidal Range Energy in Malaysia

Tidal range energy is generated from the tide-generating force, i.e., tide rise and fall influenced by the Sun and Moon system [18] and local bathymetry. A regional study stated that the Malaysian coastal area has the potential to harvest power from tidal range energy [19]. Kai et al., [7] stated that Malaysia has low tide, low current speed, and low kinetic-energy flux, which is not significant enough for device deployment, but it can be realised by improving the efficiency of the device. Previous studies have identified several locations that have the potential to harness tidal range energy. The potential location for tidal range power development can be determined by assessing the available resources based on the local tidal range and the available energy. Studies of tidal range energy potential on the east coast of Malaysia by Samo et al., [11] and [12] have identified Pending and Tanjung Manis, Sarawak, and Tawau, Sabah, are the potential sites to generate electricity of 50.7 kW, 115.4 kW, and 67.0 kW, respectively. However, both studies show discrepancies in the data observation, which is that the estimated tidal range value at Tawau has not been briefly explained since the study utilised the tidal data from the Sarawak Marine Department. Besides, the site analysis has not well presented since the location probably contains various marine conflicts. Research by Samo et al., [20] has proposed a preliminary turbine design for the existing barrage in Kuching, Sarawak. The study proposed one-way operation bulb-type turbine that can yield 10.23 GWh/year of tidal range power at Kuching barrage.

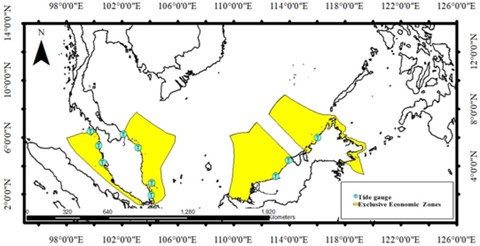
Figure 1: The tidal range energy potential assessment is conducted within the Malaysian Exclusive Economic Zone (EEZ).

Figure 2: The measurement of sea surface height from Jason-2 with respect to ellipsoid [32].

1.2 Study Area

The study area is circumscribed by latitude from 0˚ to 14˚ and longitude from 95˚ to 126˚, as shown in Figure 1. This study area focuses on the Malacca Straits, South China Sea, Celebes Sea, and Sulu Sea. The available resources and site suitability analysis is performed inside the Malaysian Exclusive Economic Zone (EEZ). According to the UN General Assembly (UNGA) [21], the EEZ extends to 200 nautical miles from the territorial baseline and stretches to 12 nautical miles of the territorial Sea. Within this area, the coastal nation has exclusive control and exploitation rights over natural resources. This zone has been documented in the Exclusive Economic Act 1984 (Act 311) of Malaysian jurisdiction. The ocean renewable energy development must be confined inside the EEZ of the marine country [22]. The WGS84 coordinate system is mainly used for the geodetic datum realisation of this study. Tide gauge data have been acquired from the Department of Survey and Mapping Malaysia (DSMM). Of 21 tide gauge stations, only 10 are used, 7 stations in Peninsular Malaysia and 3 stations in Sabah and Sarawak, as depicted in Figure 1.

2. Data and Methods

2.1 Altimetry Sea Surface Height (SSH)

The altimeter principle on sea surface height measurement is established from the time travel from and back of the altimeter radar signal towards the sea surface [23] and [24], as shown in Figure 2. Based on Figure 2, satellite altimeters measure sea surface height by emitting radar or microwave pulses and timing how long it takes for these pulses to bounce off the ocean's surface and return to the satellite.

By calculating the round-trip travel time, the satellite can determine the distance between itself and the sea surface, providing accurate measurements of sea surface height. Meanwhile, the three-dimensional position of the altimeter in relation to a fixed Earth coordinate system is determined using an independent tracking mechanism. Din et al., [25] stated that merging these two measurements (i.e., altimeter signal measurement and altimeter position) produces sea surface heights' profile or ocean level with reference to the ellipsoid. The emission of short pulse radiation and measuring the time taken of signal travelled to the sea surface and back to the satellite are called altimeter range, , which describes the satellite's height above the ocean surface. The range from satellite altimeter to sea level is expressed in Equation 1, which is based on the travel period of the signal [26]:

R_corrected = R_obs – ΔR_ion – ΔR_dry – ΔR_wet - ΔR_ssb

Equation 1

where:

R_corrected = Corrected range

R_obs = Observed range by altimeter

ΔR_ion = Ionospheric correction

ΔR_dry = Dry tropospheric correction

ΔR_wet = Wet tropospheric correction

ΔR_ssb = Sea-state bias correction

SSH can be acquired from the calculation of the corrected range, R_correctedand the satellite altitude height, H with respect to the ellipsoid. According to Fu and Cazenave [23], the relationship of range, R_corrected, altitude or orbital height, Hand measured SSH are incorporate to a reference ellipsoid, which is represent the sea surface equipotential includes the gravity effect of the Earth and centrifugal force (i.e., geoid). Various corrections are applied to improve the altimetry range estimation. The correction accounts for factors such as the ionosphere (ΔR_ion), dry troposphere (ΔR_dry), wet troposphere (ΔR_wet), and sea state bias (ΔR_ssb) as explain in Table 1. Hence, the estimation of sea surface height, h proportional to the ellipsoid with the adaption of R_corrected can be expressed in Equation 2:

h = H - R_corrected

Equation 2

According to Hamden [26], the accuracy of the satellite altitude is almost proportional to the accuracy of sea surface height measurement.

Thus, to improve the measurement of SSH as in Equation 2, [31] suggested to apply the range corrections. With range corrections, the measured SSH is spatially varied from ±100 meters with temporal variations up to ±10 meters. Yazid [27] suggested that applying the geophysical correction in SSH measurement is important. Altimetric SSH data is computed using the Equation 3 [26]:

Equation 3

where:

h^obs_ssh = Corrected sea surface height

H_alt = Satellite altimeter altitude

R_obs = Satellite altimeter range

h_dry , h_wet= Dry and wet tropospheric correction

h_ion = Ionospheric correction

h_sbb = Sea-state bias correction

h_sol = Solid earth tide correction

h_pole = Pole tide correction

h_DAC = Dynamic atmospheric correction

ε = Errors induce in satellite altimeter measurement

2.1.1 Collinear analysis

Collinear analysis is the method used to estimate the altimetric SSH data of every track similar to the reference tracks. In this study, the SSH time series from TOPography EXperiment (TOPEX) coincides with the Jason-1 time series and follows with the Jason-2 time series. This method is also applied to the Jason-1 time series and the Jason-2 time series. Meanwhile, the GEOSAT Follow-on (GFO) mission is a single mission; thus, the merging of altimetric sea surface height time series is not applicable. TOPEX class mission and GFO satellite have a distinctive repeat period of 9.91 days and 17.05 days, respectively. This matter affects the sea surface height data with tidal aliasing effect due to the long period of altimeter data [2]. The aliasing period or tidal aliasing is utilized as a variable during the tidal analysis and prediction for satellite altimetry data. The aliasing period can be estimated using Equation 4 [33]:

Equation 4

where:

T_a = Aliased period

f = Frequency of tidal component

Δs = Period sample

Table 1: Range corrections applied in satellite altimeter

2.2 Tidal Range Data Estimation

The time series of sea surface height from the altimeter is utilised to compute the amplitude and phase of the harmonic constants, i.e., M2, S2, K1, O1, N2, K2, P1, Q1, MF, MM, SSA, and SA using the harmonic analysis, as per stated by Hamden [26]. The harmonic analysis method uses Unified Tidal Analysis and Prediction Functions (UTide) software.

UTide software is fully operated in a MATLAB environment developed by Codiga [34] for tidal analysis and signal identification. The estimated twelve tidal constituents are occupied into the tidal harmonic equation used to predict the Lowest Astronomical Tide (LAT) and Highest Astronomical Tide (HAT) relative to Mean Sea Level (MSL).

In this research, at least 19 years of tide prediction are engaged to generate the LAT and HAT models by Byun et al., [35]. Hamden [2] justified that using 19 years of estimated harmonic constant from 19 years of tidal data is acceptable to produce the HAT and LAT data. The tidal range data is derived from 23 years of data of TOPEX-class mission and 8 years of GFO mission. Subsequently, tidal range data are estimated from the processed altimetric sea surface height time series data. The predicted LAT and HAT are used to calculate the value of the tidal range over the Malaysian seas. The lowest and highest astronomical tides occur under meteorological and a combination of astronomical conditions. The estimated height or astronomical tidal range between the HAT and LAT can be expressed using Equation 9:

T_range = T_HAT - T_LAT

Equation 9

Where:

T_range = Tidal range (m)

T_HAT = Highest Astronomical Tide (m)

T_LAT = Lowest Astronomical Tide (m)

2.3 Tidal Range Energy Derivation

Tidal range energy consists of potential and kinetic energy [19]. The tidal range value over the study area is derived from the tidal analysis of altimeter sea surface height data and the tide difference between the LAT and HAT, as in Equation 9. The theoretical tidal range energy density (kW/m²) can be estimated using the Equation 10 [18]:

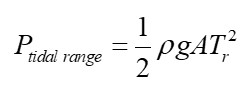
Equation 10

Where:

P_{tidal range} = Tidal range energy density (kW/m²)

ρ = Density of the seawater, 1026 kg/m³

g = Acceleration due to the Earth's gravity (9.807 m/s²)

A = Horizontal area of enclosed basin (km²)

T_r = Tidal range (m)

2.4 Data Validation

Validation plays a key role in proving the accuracy of the altimetric data. The wind speed, significant wave height and sea surface height data from the satellite altimeter are validated with the in-situ measurement. The information on selected tide gauges is tabulated in Table 2. A collocation between the altimetric and in-situ data is performed using the statistical method to interpret the relation and residuals between the data sets. The estimated tidal range data from the height difference of derived HAT and LAT relative to MSL are validated by comparing the spline interpolation (minimum spline curvature) of HAT and LAT with the local tide gauge. There are 10 selected tide gauges from the Department of Survey and Mapping Malaysia (DSMM) used to validate the HAT and LAT tidal datums, as illustrated in Figure 1. The comparison of HAT and LAT with the tide gauge is assessed using the statistical evaluation by calculating the Root Mean Square Error as in Equation 11:

Equation 11

Where:

y_i = Observed data (satellite altimeter)

x_i = Measured data (tide gauge)

N = Number of datasets

RMSE = Root Mean Square Error

Table 2: List of tide gauge stations used in this study

Table 3: Statistical results between altimetry-derived LAT (minimum spline curvature) and in situ tide gauge data

Table 4: Statistical results between altimetry-derived HAT (minimum spline curvature) and in situ tide gauge data

3. Results and Discussion

3.1 Data Validation

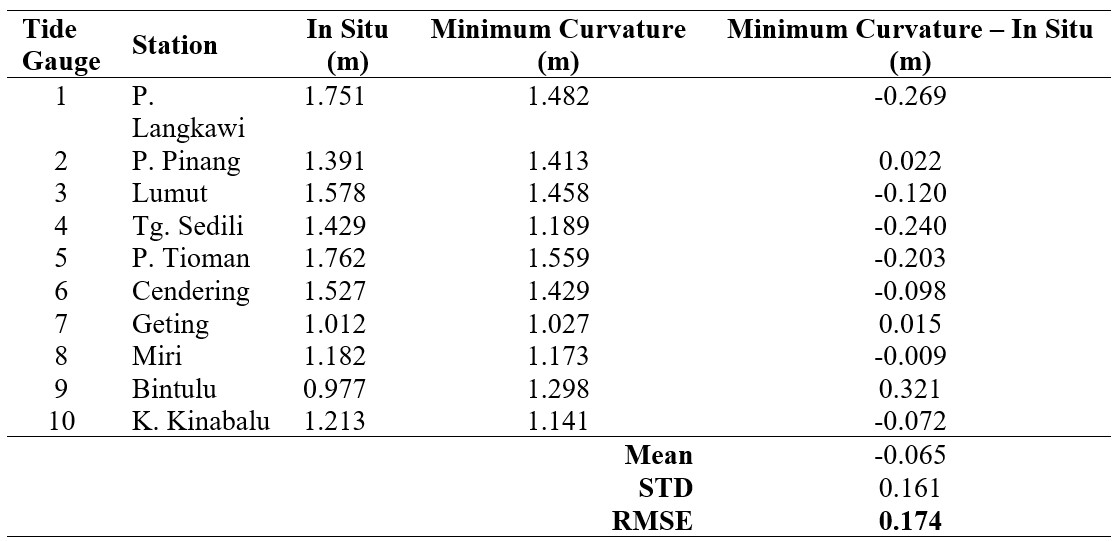
To validate tidal range data, the altimetry-derived LAT and HAT with respect to MSL were compared with 10 coastal tide gauge stations in Table 3 and Table 4, respectively. The results of the comparison, as reported by Hamden [26], indicate that the root mean square error (RMSE) values of the derived LAT and HAT with respect to MSL with the tide gauges were 17.4 centimetres (0.174 meters) and 25.5 centimetres (0.255 meters), respectively.

3.2 Tidal Range Characteristics over the Malaysian Sea

Figure 3 illustrates the average tidal range estimated from the altimetry sea surface height over the Malaysian Sea. Generally, the tidal range is the vertical difference between the high and low tide water levels in each area. As for this study, it is estimated from the HAT and LAT. As depicted in Figure 3, the tidal ranges in Malaysia vary significantly based on the location. On the east coast of Peninsular Malaysia, the tidal ranges are commonly smaller than the west coast (Malacca Straits).

Figure 3: Derived tidal range over the Malaysian Sea

The average tidal range on the east coast of Peninsular Malaysia ranges between 1.5 to 3.5 meters. Meanwhile, at the centre of Malacca Straits, the tidal range heights vary with an average of up to 5 meters. High tidal range height, with an average of 5 meters, also occurs along the coast of Sematan to Pulau Bruit of Sarawak, as shown in Figure 3.

Several factors influence the variation of tidal ranges in Malaysia. Apart from the gravitational pull of the astronomical bodies, this study has analysed that the location, the basin's characteristics, and the water's depth also affect the tidal range variation. For example, in Malacca Straits, the shape of the basin is broadened in the north and narrowed towards the south, which could lead to a faster water movement during the tidal flow, resulting in a higher tidal range in the central Malacca Straits. According to [36], the higher tidal range in Malacca Straits is due to the interaction between two tidal waves from different directions: from the northern part of Malacca Straits (Andaman Sea) and the Singaporean waters. This event causes the accumulation of M2 current with different rotations in the middle of Malacca Straits, where anticlockwise rotation along the west (near Sumatra) and clockwise rotation in the east (near the west coast of Malaysia) [36].

Furthermore, a higher tidal range with an average of up to 5 meters is recorded along the Sematan-Maludam coastlines, as in Figure 3. The basin area of these locations is close to a large river and a few minor rivers, which lead to a large volume of water flow in and out of the river, which increases the tidal range. Besides that, these areas also have shallow depths that could generate higher waves as the waves reach the shore, leading to higher tides.

According to Rijn [37], the tidal range is affected by funnelling and shoaling activities in the estuary (i.e., river). The terms funnelling and shoaling refer to the gradual reduction in width and depth of an estuary, respectively. As depicted in Figure 4, the width (red lines) of the river near Maludam, Sarawak, gradually decreases towards the land. Some coastal locations, particularly those close to river mouths and estuaries, have shallow waters where sediment from the rivers builds up and creates shallow zones [38].

Besides that, Kuala Rompin and Tioman Island coastal areas experience a tidal range average of up to 3.5 meters. According to Haditiar et al., [36], the shoaling waves around the Ho Chi Minh coast generate high M2 amplitude, which produces high tides in the coastal areas and increases M2 in Peninsular Malaysia. However, the generated tidal range on the east coast of Peninsular Malaysia is not as high as on the west coast. This is because of the geographical features on the east coast of Peninsular Malaysia, encompassed by a close to shallow, semi-enclosed basin of the Gulf of Thailand and a relatively flat ocean depth with an average of only 45 meters [39]. The flat depth of the Gulf of Thailand limits water movement, resulting in a low tidal range. Based on the finding of the tidal range characteristics in Malaysian seas, the potential locations for tidal range energy development are at the coastal areas, especially on the west coast of Peninsular Malaysia (Malacca Straits) and Sematan to Pulau Bruit (Maludam) coastlines, as tabulated in Table 5. Table 5 summarises the potential locations for tidal range energy over the Malaysian seas based on the estimated average of tidal range from the altimetry SSH data.

Table 5: Potential location for harnessing tidal range energy based on estimated tidal range from altimetry SSH data

Figure 4: Estuary width on the river near the Maludam coastlines of Sarawak (retrieved from Google Earth). The width of the estuary decreases (red arrows) towards the land and creates funnelling and shoaling activities, which affect the dynamic of the tidal range

Figure 5: Resource map for tidal range energy in EEZ (kW/m²)

3.3 Tidal Range Energy Resource Assessment

The indication of potential location based on the tidal range energy resource is analysed using the Geographical Information System (GIS). The assessment is conducted inside the EEZ, divided into 60 zones, with 10 in the Malacca Straits, 14 on the east coast of Peninsular Malaysia, 21 in the Sarawak Sea and 15 in the Sabah Sea. Based on Figure 5, most of the available tidal range energy in Malaysia is concentrated in the coastal region.

Table 6: Potential location for harnessing tidal range energy inside EEZ

This study found that Malacca Straits, Perlis and Kedah coastlines, Langkawi and Dayang Bunting Island, and Sarawak region, especially along the Tanjung Datu to Matu coastlines, have the highest tidal range energy density range from 58.32 kW/m² to 129.33 kW/m², as shown in Figure 5. Thus, 8 zones are identified as potential areas, including K1, K2, K3, K4, M2, M3, M9 and M10. K1 and K2 zones located between Sematan and Kabong, Sarawak, have the highest average tidal energy of 93.33 kW/m² and 120.96 kW/m², with average tidal range height of 4.29 meters and 4.90 meters, respectively.

Meanwhile, Malacca Straits (M10) is estimated at 89.14 kW/m², the highest available resource for Peninsular Malaysia. According to [12], Pending and Tanjung Manis in Sarawak have the potential for tidal range energy development, generating tidal power up to 33.1 kW and 50.7 kW, respectively. As for this study, both Pending and Tanjung Manis, Sarawak, are located inside the K1 and K2 zones, respectively, and these zones are considered the most potential for tidal range energy development. Table 6 summaries the potential location for tidal range energy inside the Exclusive Economic Zone (EEZ).

3.4 Technology for Tidal Range Energy Resources

The tidal range is extracted from the tide height difference between low and high tide. The concept of a tidal range power plant is to impound a large volume of water in an area where a head difference can be created and then let the water flow in or out of this area through low-head hydrokinetic turbines [6]. The tidal range is predictable and not affected by the weather conditions but rather by the gravity of the celestial bodies, including the moon, sun, and Earth [40]. Kempener and Neumann [40] have summarised the options of tidal range technology for power generation. Firstly, one-way power generation at ebb tide is shown in Figure 6(a). This scheme operates when the reservoir is filled at the flood tide through sluice gates or closed valves once the tide has reached its highest level. During the ebb tide, the power is generated once the water in the reservoir is released through turbine. Secondly, one way power generation during the flood tide as in Figure 6(b). One way power generation during the flood tide operates when the sluice gates are kept closed to isolate the reservoir while at its lowest level. When the tide is high, the water from the ocean flows into the reservoir via the turbines and generates power. Thirdly, is two-way power generation which both incoming and outgoing tides generate power through the turbine. This technology requires a reversible turbine to operate and generate electricity.

A study by [42] determined six locations with high tidal ranges for coastal regions, including Sejingkat, Pelabuhan Klang, Pulau Langkawi, Tawau, Kukup, and Johor Bahru. This result agrees with this study, as illustrated in Figure 5. According to [43], Pelabuhan Klang in Malacca Straits has fulfilled the minimum requirement for tidal barrage development with a tidal height of more than 3 meters, while Sabah and Sarawak have the highest tide range with 5 meters. Samo et al., [44] indicated that the Kuching Barrage installed near the Sarawak River, which operates to mitigate flood in Kuching, has the potential to be developed into a tidal range power plant as its highest tidal range of up to 6.8 meters. Samo et al., [44] also proposed a tidal power plant scheme (Figure 7) that could be operated in Kuching Barrage using one-way power generation during the ebb tide, generating 20.17 MW daily and 35.41 GWh per year. It is clearly stated that the existing barrage in Malaysia can harness the tidal range power by just designing and installing a suitable turbine that meets the requirements, such as a minimum of 3 meters and 5 meters of tidal height and tidal range, respectively.

Figure 6: Tidal barrage operation for one-way power generation during ebb tide(a) and during flood tide (b) [41]

Figure 7: The cross-section of Kuching Barrage with the proposed of bulb type turbine for power generation during the ebb tide [44]

Even though Malaysia has a low tidal range compared to other coastal countries that utilise tidal range for electricity generation, a small tidal barrage is more desirable as it requires less monetary and is more manageable than a large tidal barrage [19]. Besides that, the location for tidal barrage development should avoid the marine ecosystem, such as the movement of fish in and out of the estuaries, and the socio-economic, such as the fishing area and the passage of fishing boats.

4. Conclusion

The escalating impact of climate change and the depletion of natural resources has intensified the need for clean and sustainable energy sources, particularly ocean renewable energy. In this study, satellite altimeter data is employed to identify suitable areas for ocean renewable energy in Malaysia due to the limited availability of in-situ measurements for assessing potential locations.

Hence, this study leverages satellite altimeter data, which offers extensive spatial and temporal coverage, efficient data collection, and sufficient accuracy in retrieving essential ocean parameters for analyzing the potential of tidal range energy in Malaysian waters. This study demonstrates that satellite altimeter data provides reliable tidal range data. Tidal height range characteristics in Malaysia increase as the Sea approaches the shoreline, but this is predominantly observed in several locations that experience predominantly semidiurnal tides. These locations include Kedah, Perlis, and Penang nearshore areas, Rompin, Pahang, the centre of the Malacca Straits, and Sarawak along the Sematan to Bruit Island stretch. Regarding tidal range resource, 9 potential zones are identified, with zone K2 in the Sarawak coastal area displaying a potential tidal range energy density ranging from 108.14 kW/m² to 129.33 kW/m² and an average tidal range of 4.90 meters.

This study provides information on the available technologies that can serve as a reference for designing suitable energy-harnessing systems in the Malaysian seas. Existing technologies are primarily optimized for higher-resource regions, particularly in European countries. However, there is a significant opportunity for deployment if devices can be optimized specifically for lower energy resource regions like Malaysia. Although this study does not include a detailed technical assessment, the information presented here is a valuable insight for future technological development in ocean renewable energy.

Acknowledgements

The authors thank TU Delft Altimetrics LLC for providing altimetry data through the Radar Altimeter Database System (RADS) and the Department of Survey and Mapping Malaysia for providing the tide gauge data. This project is funded by the Ministry of Higher Education (MOHE) under the Fundamental Research Grant Scheme (FRGS) Fund, Reference Code: FRGS/1/2021/WAB05/UTM/02/1 (UTM Vote Number: R.J130000.7852.5F478).

References

[1] Dipper, F., (2022). The Seawater Environment and Ecological Adaptations. Elements of Marine Ecology (5th ed.,). F. Dipper, Ed. Butterworth-Heinemann, 37-151. https://doi.org/10.1016/B978-0-08-102826-1.00002-8.

[2] Hamden, M. H., Din, A. H. M., Wijaya, D. D. and Pa’suya, M. F., (2021). Deriving Offshore Tidal Datums Using Satellite Altimetry Around Malaysian Seas, in IOP Conf. Ser. Earth Environ. Sci. Proc. of the 4th International Conference on Science and Technology Applications in Climate Change, Vol. 880, https://doi.org/10.1088/1755-1315/880/1/012011.

[3] NOAA, (2023). What is a Tide Gauge? [Online]. Available: https://oceanservice.noaa.gov/about/faq.html. [Accessed Jul. 5, 2023].

[4] Selin, N. E., (2023). Tidal Power. Encyclopedia Britannica , Dec. 15, 2022. [Online]. Available: https://www.britannica.com/science/tidal-power. [Accessed Jun. 18, 2023].

[5] McErlean, T., (2007). Harnessing the Tides, The Early Medieval Tide Mills at Nendrum Monastery, Strangford Lough, Northern Ireland Archaeological Monographs No 7 . The Stationery Office.

[6] Greaves, D. and Iglesias, G., (2018). Wave and Tidal Energy , 1st ed. Hoboken: John Wiley & Sons, Ltd, [E-book] Available: Wiley Online Library.

[7] Lim, Y. K., Sarip, S., Kaidi, H. M., Rey, J. A. A., Samsuddin, N. M., Muhtazaruddin, M. N., Sukki, F. M., Aziz, S. A. (2021). Current Status and Possible Future Applications of Marine Current Energy Devices in Malaysia: A Review, IEEE Access, Vol. 9, https://doi.org/10.1109/ACCESS.2021.3088761.

[8] Roberts, A., Thomas, B., Sewell, P., Khan, Z., Balmain, S. and Gillman, J., (2016). Current Tidal Power Technologies and their Suitability for Applications in Coastal and Marine Areas. J. Ocean Eng. Mar. Energy , Vol. 2, 227-245. https://doi.org/10.1007/s40722-016-0044-8.

[9] Rahim, M. W. A., Rahman, A. A., Izham, M. and Amin, N. A. M., (2023). Tidal Energy in Malaysia: An Overview of Potentials, Device Suitability, Issues and Outlook. Reg. Stud. Mar. Sci., Vol. 61, https://doi.org/10.1016/j.rsma.2023.102853.

[10] Shafie, S. M., Mahlia, T. M. I., Masjuki, H. H. and Andriyana, A., (2011). Current Energy Usage and Sustainable Energy in Malaysia: A Review. Renew. Sustain. Energy Rev., Vol. 15(9), 4370-4377. https://doi.org/10.1016/j.rser.2011.07.113.

[11] Samo, K. A., Rigit, A. R. H. and Baharun, A., (2017). Mapping of Tidal Energy Potential based on High and Low Tides for Sabah and Sarawak. MATEC Web Conf., Vol. 87, https://doi.org/10.1051/matecconf/20178702007.

[12] Samo, K. A., Samo, I. A., Siyal, Z. A. and Rigit, A. R. H., (2020). Determination of Potential Tidal Power Sites at East Malaysia. Eng. Technol. Appl. Sci. Res., Vol. 10(4), 6047-6051.
https://doi.org/10.48084/etasr.3674.

[13] Nerem, R. S. and Mitchum, G. T., (2001). Chapter 6 Observation of Sea Level Change from Satellite Altimetry. Sea Level Rise, Vol. 75, 121–163. https://doi.org/10.1016/S0074-6142(01)80009-7.

[14] Vignudelli, S. and De Biasio, F., (2021). Coastal Sea Level Trends from a Joint Use of Satellite Radar Altimetry, GPS and Tide Gauges: Case Study of the Northern Adriatic Sea. Geodetic Sciences- Theory, Applications and Recent Developments. 1-29. https://doi.org/10.5772/intechopen.98243.

[15] Nehama, F. P. J., Veriua, Z. D. H., Maueua, C., Hibbert, A., Calafat, F. and Cotton, P. D., (2022). Validating Sea-Level Altimetry Data against Tide Gauge for Coastal Risk Analysis in Mozambique. J. Mar. Sci. Eng. , Vol. 10(10). https://doi.org/10.3390/jmse10111597.

[16] Hamden, M. H., Din, A. H. M. and Wijaya, D. D., (2021). A Comparison between Tidal Constituents Derived from Satellite Altimetry and In-Situ Data Around Peninsular Malaysia. J. Inf. Syst. Technol. Manag. , Vol. 6(24), 139-151. https://doi.org/10.35631/JISTM.624015.

[17] Alifdini, I., Kismawardhani, R. A., Wirasatriya, A., Sugianto, D. N., Yaakob, O. and Widodo, A. B., (2018). Identification of Tidal Energy Resources using Satellite Altimetry Data for Indonesian Seas. 4th International Symposium on Geoinformatics (ISyG), 1-6. https://doi.org/10.1109/ISYG.2018.8611798.

[18] Neill, S. P., Angeloudis, A., Robins, P. E., Walkington, I. A., Ward, S. L., Masters, I., Lewis, M. J., Piano, M., Avdis, A., Piggott, M. D., Aggidis, G. A., Evans, P., Adcock, T. A., Židonis, A., Ahmadian, R. and Falconer, R. A., (2018). Tidal Range Energy Resource and Optimization – Past Perspectives and Future Challenges. Renewable Energy. Renew. Energy, Vol. 127, 763-778. https://doi.org/10.1016/j.renene.2018.05.007.

[19] Nazri, N., Anuar, S., Basrawi, F., Shukrie, A. and Aishah, S., (2018). Evaluation of Potential Sites for Harnessing Tidal Energy around Coastal Area of Malaysia. MATEC Web Conf., Vol. 225, 1-10. https://doi.org/10.1051/matecconf/201822506015.

[20] Samo, K. A., Baharun, A. and Rigit, A. R. H., (2021). The Development of a Preliminary Design for a Tidal Energy Plant. Eng. Technol. Appl. Sci. Res. , Vol. 11(1), 6730–6733. https://doi.org/10.48084/etasr.3980.

[21] UNGA, (1982). United Nation Convention on the Law of the Sea (UNCLOS). [Online]. Available: https://www.un.org/Depts/los/convention_agreements/convention_overview_convention.htm. [Accessed March 02, 2023].

[22] Elsner, P. and Suarez, S., (2019). Renewable Energy from the High Seas: Geo-spatial Modelling of Resource Potential and Legal Implications for Developing Offshore Wind Projects Beyond the National Jurisdiction of Coastal States. Energy Policy, Vol. 128, 919-929. https://doi.org/10.1016/j.enpol.2019.01.064.

[23] Fu, L. L. and Cazenave, A., (2001). Satellite Altimetry and Earth Sciences (Ed., 1st). Academic Press, https://doi.org/10.1016/S0074-6142(01)80145-5.

[24] Din, A. H. M., (2014). Sea Level Rise Estimation and Interpretation in Malaysian Region Using Multi-Sensor Technique . Ph.D Dissertation, Universiti Teknologi Malaysia, Johor Bahru, Johor, Malaysia.

[25] Din, A. H. M., Ses, S., Omar, K. M., Naeije, M., Yaakob, O. and Pa'suya, M. F., (2014). Derivation of Sea Level Anomaly Based on the Best Range and Geophysical Corrections for Malaysian Seas using Radar Altimeter Database System (RADS). J. Teknol., Vol. 71(4). https://doi.org/10.11113/jt.v71.3830.

[26] Hamden, M. F., (2022). Development of Quasi-Seamless Hydrographic Separation Models Based on Satellite Altimetry and Coastal Tide Gauges in Malaysia . Ph.D Dissertation, Universiti Teknologi Malaysia, Johor Bahru, Johor, Malaysia.

[27] Yazid, N., (2018). Marine Geoid Determination over Malaysian Seas Using Satellite Altimeter and Gravity Missions . MPhil Dissertation, Universiti Teknologi Malaysia, Johor Bahru, Johor, Malaysia.

[28] Saastamoinen J., (2013). Atmospheric Correction for the Troposphere and Stratosphere in Radio Ranging Satellites. The Use of Artificial Satellites for Geodesy , Vol. 15, 247–251. https://doi.org/10.1029/GM015p0247.

[29] Cretaux, J. F., Nielsen, K., Frappart, F., Papa, F., Calmant, S. and Benveniste, J., (2017). Hydrological Applications of Satellite AltimetryRivers, Lakes, Man-Made Reservoirs, Inundated Areas. Satellite Altimetry over Oceans and Land Surfaces , 459–504. https://doi.org/10.1201/9781315151779-14.

[30] Gaspar, P. and Florens, J. P., (1998). Estimation of the Sea State Bias in Radar Altimeter Measurements of Sea Level: Results from a New Nonparametric Method. J. Geophys. Res. Ocean., Vol. 103,
https://doi.org/10.1029/98JC01194.

[31] Andersen, O. B. and Scharroo, R., (2011). Range and Geophysical Corrections in Coastal Regions: and Implications for Mean Sea Surface Determination. Coastal Altimetry. 103–145. https://doi.org/10.1007/978-3-642-12796-0_5.

[32] JPL, (2022). Ocean Surface Technology from Space, Jet Propulsion Laboratory . [Online]. Available: https://sealevel.jpl.nasa.gov/missions/technology/. [Accessed Aug. 26, 2022].

[33] Lindsley, R. D., (2013). Fitting Tidal Constituents to Altimeter Data. [Online]. Available: https://digitalcommons.usu.edu/cgi/viewcontent.cgi?referer=&httpsredir=1&article=1070&context=spacegrant.

[34] Codiga, D. L., (2011). Unified Tidal Analysis and Prediction Using the UTide Matlab Functions . Graduate School of Oceanography, Narragansett, Rhode Island, USA, Tech. Rep. 2011-01.

[35] Byun, D. S. and Hart, D. E., (2019). On Robust Multi-Year Tidal Prediction Using T_TIDE. Ocean Sci. J., Vol. 54(4), 657-671. https://doi.org/10.1007/s12601-019-0036-4.

[36] Haditiar, Y., Putri, M. R., Ismail, N., Muchlisin, Z. A., Ikhwan, M. and Rizal, S., (2020). Numerical Study of Tides in the Malacca Strait with a 3-D Model. Heliyon, Vol. 6(9), https://doi.org/10.1016/j.heliyon.2020.e04828.

[37] van Rijn, L. C., (2011). Analytical and Numerical Analysis of Tides and Salinities in Estuaries; Part I: Tidal Wave Propagation in Convergent Estuaries. Ocean Dynamics, Vol. 61(11), 1719-1741. https://doi.org/10.1007/s10236-011-0453-0.

[38] Short, A. D., (2012). Coastal Process and Beaches. Nat. Educ. Knowl. , Vol. 3(10). https://www.nature.com/scitable/knowledge/library/coastal-processes-and-beaches-26276621/.

[39] Lange, I. D., Schoenig, E. and Khokiattiwong, S., (2019). Thailand, World Seas: An Environmental Evaluation, 2nd ed., C. Sheppard, Ed. Elsevier, 491–513.

[40] Kempener, R. and Neumann, F., (2014). Tidal Energy Technology Brief. International Renewable Energy Agency. [Online]. Available: https://www.irena.org/Publications/2014/Jun/Tidal-Energy.

[41] Yusoff, N. A. M., Ramli, N. L. and Mohamed, M. R., (2015). Investigation of the Potential Harnessing Tidal Energy in Malaysia. ARPN J. Eng. Appl. Sci., Vol. 10(21), 9835–9841.
https://www.arpnjournals.org/jeas/research_papers/rp_2015/jeas_1115_2992.pdf.

[42] Lee, K. S. and Seng, L. Y., (2013). Simulation Studies on the Electrical Power Potential Harnessed by Tidal Current Turbines. J. Energy Environ. , Vol. 1, 18-23. https://journal.uniten.edu.my/index.php/jee/article/view/88/83.

[43] Nazri, N., Anuar, S., Basrawi, F., Shukrie, A. and Aishah, S., (2016). Estimation of Energy Potential and Power Generation from Tidal Basin in Coastal Area of Malaysia. MATEC Web Conf., Vol. 38, https://doi.org/10.1051/matecconf/20163802008.

[44] Samo, K. A., Rigit, A. R. H., Samo, I. A., Bukhari, A. A. S., Soomro, A. and Baharun, A., (2017). Suitable Powerhouse Design for Kuching Barrage Tidal Power Scheme. Int. J. Eng. Sci. Res. Technol. , Vol. 6, https://doi.org/10.5281/zenodo.886756.